
 |
Exam-Style Questions.Problems adapted from questions set for previous Mathematics exams. |
1. | IB Studies |

The diagram show a wheelchair ramp from ground level to a height of 70cm.
(a) Calculate the gradient of the ramp.
Ramp manufacturers recommend a gradient no steeper than 1:12 for safe independent use.
(b) Using your answer to part (a), comment on whether this wheelchair ramp is safe for independent use.
The equation of a different wheelchair ramp is \(2x + 22y -1860 = 0\) where \(y\) represents the height and \(x\) the horizontal distance, both in centimetres.
(c) Find the horizontal distance covered by this wheelchair ramp.
(d) Determine whether this wheelchair ramp is safe for independent use.
2. | IB Standard |
Percy Cod and Fran Finklestein are both researchers working at different universities. They are each studying a different colony of bacteria which coincidentally start increasing in size at the same time.
The number of bacteria in Percy's colony, after \(t\) hours, is modelled by the function \(P(t)=8e^{0.3t}\).
(a) Find the initial number of bacteria in Percy's colony.
(b) Find the number of bacteria in Percy's colony after four hours.
(c) How long does it take for the number of bacteria in Percy's colony to reach 350?
The number of bacteria in Fran's colony, after t hours, is modelled by the function \(F(t)=16e^{kt}\).
(d) After four hours, there are 35 bacteria in Fran's colony. Find the value of \(k\).
(e) The number of bacteria in Percy's colony first exceeds the number of bacteria in Fran's colony after \(n\) hours, where \(n\in \mathbb Z\). Find the value of \(n\).
3. | IB Studies |
The cross-section of a fish pond is drawn on a set of axes shown below. The edge is modelled by \(y=ax^2+c\) and the cross section is the same for the whole of its length. The curve touches the x-axis at the origin.
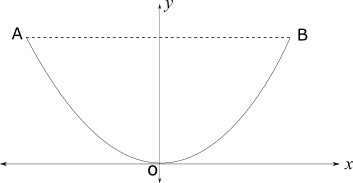
Point A has coordinates (-9,5.4) and point B has coordinates (9,5.4).
(a) Find the value of \(c\).
(b) Find the value of \(a\).
(c) Hence write down the equation of the quadratic function which models the edge of the fish pond.
(d) Calculate the value of \(y\) when \(x\)=7.2m.
(e) State what the value of \(x\) and the value of \(y\) represent for this fish pond.
(f) Find the value of \(x\) when the height of water in the pond is 2m.
The pond is filled so that the cross-sectional area of the water is 22.9m2. The pond has a length of 8m.
(g) Calculate the volume of water in the pond.
4. | GCSE Higher |
The quantity of heat required to heat an amount of water is given by the formula:
$$H = atI^2 – b$$Where \(H\) is the number of calories delivered by an electric current of \(I\) amps acting for \(t\) seconds and \(a\) and \(b\) are constants.
(a) Rearrange the formula to make \(I\) the subject.
The graph below gives information about the cooling of a cup of coffee on a cold day. The vertical axes shows the variation in the temperature, \(T\), and the horizontal axis shows the time, \(t\), in seconds.
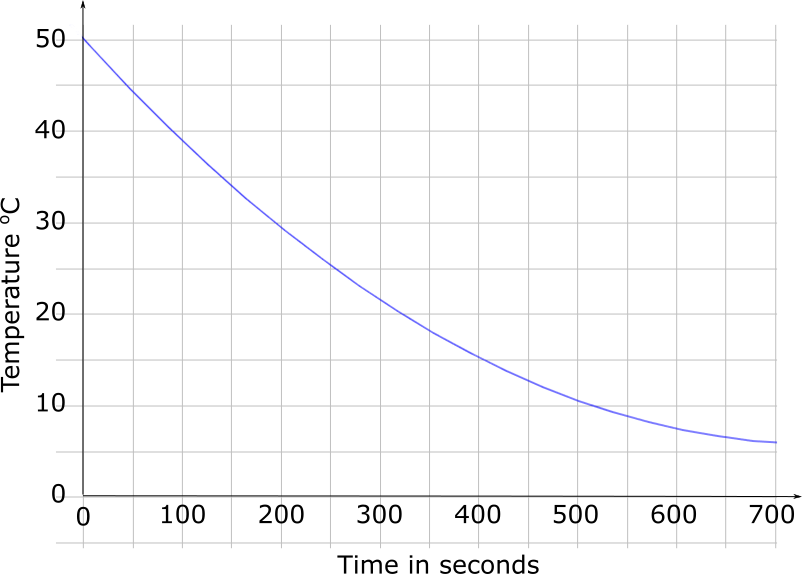
(b) Work out the average rate of decrease of the temperature of the coffee between \(t = 0\) and \(t = 700\).
The instantaneous rate of decrease of the temperature of the water at time \(A\) seconds is equal to the average rate of decrease of the temperature of the water between \(t = 0\) and \(t = 700\).
(c) Find an estimate for the value of \(A\) showing how you got your answer.
5. | IB Applications and Interpretation |
In a fantasy story the power value of a dream catcher varies depending on its length. The power values of various dream catchers are recorded in the following table:
| Length, \(x\) cm | 0 | 10 | 15 |
| Power, \(p\) W | 0 | 12 | 22 |
This information was used to create Model A, where \(p\) is a function of \(x\) , \(x \ge 0\).
Model A: \(p(x) = ax^2 + bx\) , where \(a,b \in \mathbb{Z}\).
When the length is 10 cm, Model A can be represented by the equation 50a + 5b = 6.
(a) Write down a second equation to represent Model A, when the length is 15cm.
(b) Find the values of a and b.
(c) Find the coordinates of the vertex of the graph of \(y = p(x)\).
(d) Using the values in the table and your answer to part (c), sketch the graph of \(y = p(x)\) for \(0 \le x \le 15\) and \(0 \le p \le 22\).
Additional data was used to create Model B, a revised model for the power of a dream catcher.
Model B: \(p(x) = 0.06x^2 + 0.68x\)
(e) Use Model B to calculate an estimate for the power of a dream catcher of length 18cm.
The actual power of a dream catcher of length 18cm is 30 W.
(f) Calculate the percentage error in the estimate in part (e).
6. | IB Studies |
A cup of hot tea is placed on a table to cool. The temperature of the room is 24oC.
This situation can be modelled by the exponential function:
$$T = a + b (k^{-m})$$where \(T\) is the temperature of the water, in oC, and \(m\) is the number of minutes for which the cup has been placed on the table.
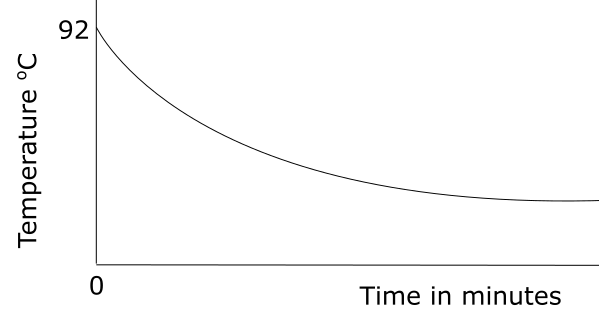
(a) Find the value of \(a\)
Initially the temperature of the tea is 92oC.
(b) Find the value of b.
After one minute, the temperature of the tea is 74oC.
(c) Find the value of \(k\).
(d) Find the temperature of the tea three minutes after it has been placed on the table.
(e) Find the total time needed for the tea to reach a temperature ofof 30oC. Give your answer in minutes and seconds, correct to the nearest second.
7. | IB Standard |
Fifty milligrams of a certain type of medicine has been shown to enter the bloodstream of an adult according to the following equations:
where \(t\) is measured in minutes and \( t\ge 0 \)
(a) State the initial rate at which the medicine enters the bloodstream.
(b) Calculate the time it takes before the rate at which the medicine enters the bloodstream is halved.
(c) Find the rate of the medicine entering the bloodstream when there is 25 mg present in the bloodstream.
(d) Sketch both R and A on the same axes. Indicate clearly any intercepts and the equations of the horizontal asymptotes.
(e) Will the patient ever feel the full effect of the 50-milligram dose?
If you would like space on the right of the question to write out the solution try this Thinning Feature. It will collapse the text into the left half of your screen but large diagrams will remain unchanged.
The exam-style questions appearing on this site are based on those set in previous examinations (or sample assessment papers for future examinations) by the major examination boards. The wording, diagrams and figures used in these questions have been changed from the originals so that students can have fresh, relevant problem solving practice even if they have previously worked through the related exam paper.
The solutions to the questions on this website are only available to those who have a Transum Subscription.
Exam-Style Questions Main Page
To search the entire Transum website use the search box in the grey area below.
Do you have any comments about these exam-style questions? It is always useful to receive feedback and helps make this free resource even more useful for those learning Mathematics anywhere in the world. Click here to enter your comments.