
 |
Mystic RoseInvestigate the properties of the famous Mystic Rose using this interactive diagram |
This is a Mystic Rose. The vertices are equally spaced around the circle. You can change the number of vertices to make different Mystic Roses
Vertices:
Why is the rose mystic? You can find out by doing the investigation below.
Also below are controls for changing the colours of the lines in your Mystic Rose. Have fun!
InstructionsUse the drop-down box above to create Mystic Roses with different numbers of vertices. For each Rose you create count the number of vertex to vertex lines and count the number of regions the circle is divided into. Complete the table above with your findings. Use the 'Check' button to ensure you are counting accurately. Can you see any patterns in the results? You may be surprised to see that the number of regions in the six vertex Rose does not follow the pattern the previous Roses did. Have you any idea why? Leave a comment below if you have any thoughts. If you are keen to investigate more you may like to consider the number of crossing points! When you have completed the table you may want to print out this page and paste it into your exercise book. If you keep your work in an ePortfolio you could take a screen shot of your answers and paste that into your Maths file. |
||||||||||||||||||||||||||||||||||||||||||
|
|
||||||||||||||||||||||||||||||||||||||||||
Transum.orgThis web site contains hundreds of free mathematical activities for teachers and students. Click here to go to the main page which links to all of the resources available. Please contact us if you have any suggestions or Questions.
|
More Activities: |
|||||||||||||||||||||||||||||||||||||||||
AnswersThere are answers to this exercise but they are only available to teachers who have subscribed to Transum and are currently signed in on this computer. A Transum subscription unlocks the answers to most of the student online exercises, quizzes and puzzles. It also provides the teacher with access to quality external links on each of the Transum topic pages so that teachers can easily find the excellent resources we have found and add to the collection themselves. Class lists, lesson plans and assessment data can also be stored in the Class Admin application and the teacher also has access to the Transum Trophies earned by class members. |
||||||||||||||||||||||||||||||||||||||||||
Colour ChoiceThe following drop-down boxes allow you to customise the colours used in your Mystic Rose. The first box controls the lines joining each vertex to the adjactent vertices. The second drop-down controls the lines connecting every second vertex and so on. |
||||||||||||||||||||||||||||||||||||||||||
|
Do you have any comments? It is always useful to receive feedback and helps make this free resource even more useful for those learning Mathematics anywhere in the world. Click here to enter your comments. Printable sheets of Mystic Roses are available for use in the classroom. The first two pages have the first 12 Mystic Roses and the third page has multiple copies of the six-vertex Mystic Rose. The latter has been used successfully in shape finding investigations eg. "How many different shapes can be found in the six-vertex Mystic Rose? Can you mark the parallel sides, equal angles etc. |
||||||||||||||||||||||||||||||||||||||||||
Other shape counting starters:
How Many Squares 1?
| How Many Squares 2?
How Many Triangles 1?
|
How Many Triangles 2? | How Many Triangles 3?
How Many Rectangles?
| Rectangles Investigation |
Icosahedron |
Triangles in Hexagon |
Mystic Rose
© Transum Mathematics 1997-2025
Scan the QR code below to visit the online version of this activity.
https://www.Transum.org/go/?Num=476
From Wilipedia:
"In the mathematical field of graph theory, a complete graph is a simple undirected graph in which every pair of distinct vertices is connected by a unique edge. Drawings of complete graphs, with their vertices placed on the points of a regular polygon, appeared in the 13th century, in the work of Ramon Llull. Such a drawing is sometimes referred to as a mystic rose."

This interactive page provides a starting point for your investigation of Mystic Roses. Is there a pattern in the number of chords, regions or chord crossing points for the sequences of Roses with more and more vertices?
Close

To help you count the regions, copy the diagram into your painting program and use the flood fill tool to mark the regions when you have counted them.
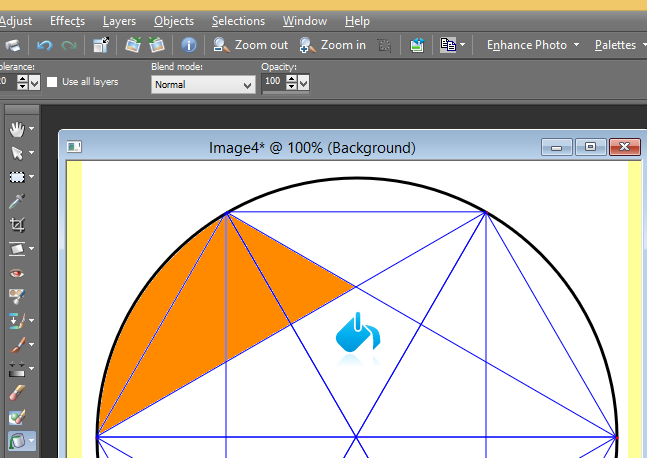
Don't wait until you have completed the table before you click on the 'Check' button. Click it often as you work through the investigation to see if you are finding the correct values.
Close

Transum,
Monday, April 20, 2015
"When considering the number of regions for the six-vertex rose it is worthwhile to draw the rose using compasses, marking of the six equally spaced points using the compasses still at the same radius then colour in the regions using only two colours and seeing the connection with the Two Colour Theorem for colouring maps.
When that is complete an extension activity could be identifying all of the shapes that can be found in the six-vertex Mystic Rose. The list will include rhombus, kite, segment, sector and many more."
@MissingPercent1, Twitter
Tuesday, November 28, 2023