Octagon Loops

Investigate the features of loops created from octagons.

Tap or click then drag from the pile of green octagons to create clones. Arrange them to create loops. Only one space is allowed inside the loop and edges should line up exactly. Each octagon touches exactly two others.

For each loop keep a record of the number of octagons, the length of the inside perimeter and the length of the outside perimeter.

Is there a connection between the number of octagons in a loop and the two perimeters?

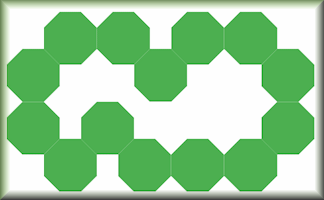
An example of an octagon loop

Extend your investigation to consider loops of other polygons. Present your findings in a way that other students of your age would understand and find interesting.

Here are some other investigations:

Suggested Leapfrog An investigation of the minimum number of moves required to make the blue and green frogs swap places. The short web address is: Transum.org/go/?to=leapfrog

Suggested Tower of Hanoi Move the pieces of the tower from one place to another in the minimum number of moves. The short web address is: Transum.org/go/?to=hanoi

Suggested Trapezia Which numbers can be represented by groups of circles arranged in the shape of a trapezium? The short web address is: Transum.org/go/?to=trapezia

... and there are many more on the Investigations page.

 

 

 

Teachers' notes for this and other Transum puzzles, exercises and activities are available here when you are signed in to your Transum subscription account. If you do not yet have an account and you are a teacher, tutor or parent you can apply for one by completing the form on the Sign Up page.

A Transum subscription also gives you access to the 'Class Admin' student management system, downloadable worksheets, many more teaching resources and opens up ad-free access to the Transum website for you and your pupils.

Register for a Transum account

This activity was originally developed in England in 1992 by SEAC (the national assessment agency) as an assessment tool for students.

Do you have any comments? It is always useful to receive feedback and helps make this free resource even more useful for those learning Mathematics anywhere in the world. Click here to enter your comments.