Here are some specific activities, investigations or visual aids we have picked out. Click anywhere in the grey area to access the resource.
What is e? Dr James Grime discusses e - the famed Euler's Number.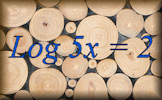
Logarithms Self-marking exercises on evaluating logarithms and using them to solve equations.
Here are some exam-style questions on this statement:
- "Find the value of the following:" ... more
- "Evaluate the following, giving your answers as integers." ... more
- "Find the value of" ... more
- "(a) Solve \(4x^2 - 8x - 5 = 0\)" ... more
- "Part of the graph of \(f(x) = {\log _b}(x + 4)\) for \(x > - 4\) is shown below." ... more
- "An arithmetic sequence has \(u_1 = \log_h(j)\) and \(u_2 = \log_h(jk)\), where \(h > 1\) and \(j, k \gt 0\)." ... more
- "Consider the function \(f (x) = \log_p(24x - 18x^2)\) , for \(0 \lt x \lt 1\), where \(p \gt 0\)." ... more
Click on a topic below for suggested lesson Starters, resources and activities from Transum.
Furthermore
Logarithms are a mathematical concept that provides a way to work with exponential equations in a more linear manner. The logarithm of a number to a given base is the power or exponent to which the base must be raised to produce that number. The most commonly used bases in logarithms are 10, often referred to as the common logarithm, and \( e \), the base of natural logarithms. The number \( e \) is an irrational number approximately equal to 2.71828 and has profound significance in mathematics, especially in calculus and complex analysis.
The key formulae for logarithms with base 10 and \( e \) are:
$$ \log_{10}(x) = y \quad \text{means} \quad 10^y = x \\ \log_e(x) = \ln(x) = y \quad \text{means} \quad e^y = x \\ \log_b(m \times n) = \log_b(m) + \log_b(n) \\ \log_b\left(\frac{m}{n}\right) = \log_b(m) - \log_b(n) \\ \log_b(m^n) = n \times \log_b(m) $$
This video on Exponential and Logarithmic Functions is from Revision Village and is aimed at students taking the IB Maths AA SL/HL course
This video on Exponential and Logarithmic Functions is from Revision Village and is aimed at students taking the IB Maths AA Standard level course.
How do you teach this topic? Do you have any tips or suggestions for other teachers? It is always useful to receive feedback and helps make these free resources even more useful for Maths teachers anywhere in the world. Click here to enter your comments.
