Here are some specific activities, investigations or visual aids we have picked out. Click anywhere in the grey area to access the resource.
Here is an Advanced Starter on this statement:
Click on a topic below for suggested lesson Starters, resources and activities from Transum.
Furthermore
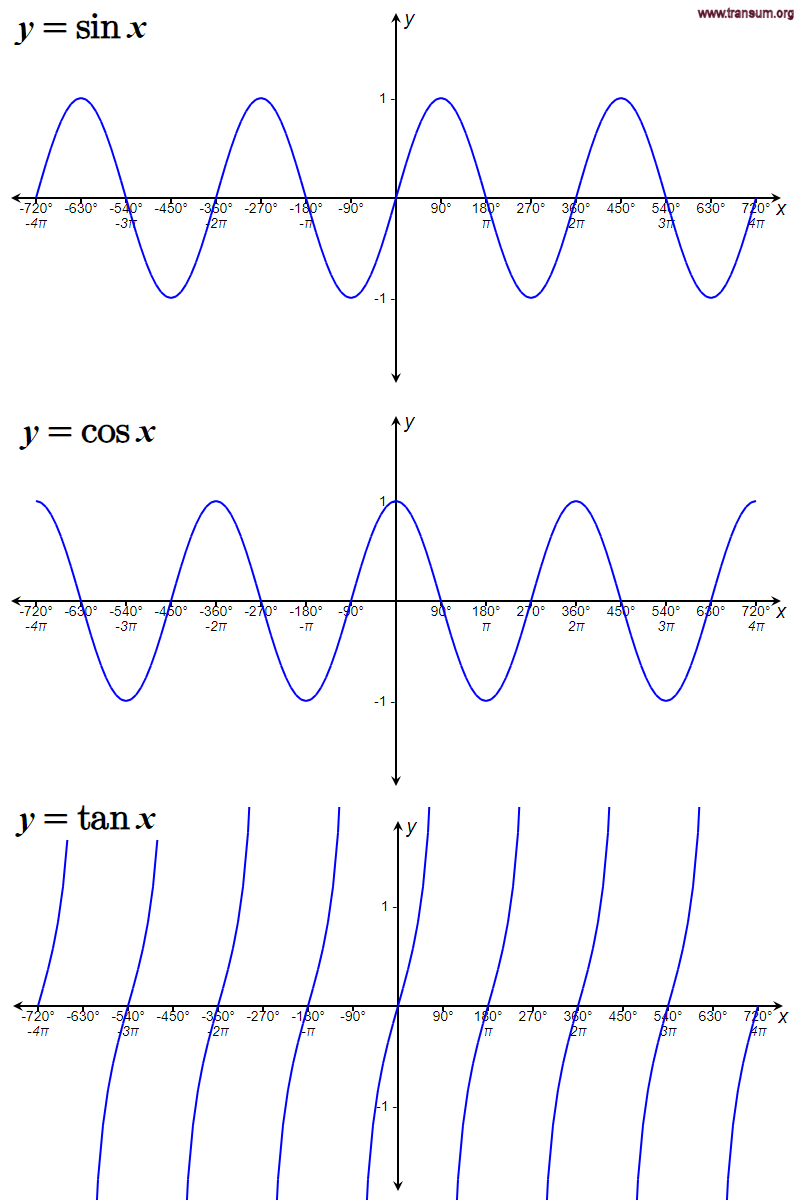
The trigonometric functions sine, cosine, and tangent exhibit distinct symmetry properties that are reflected in their graphs. The sine and cosine functions are periodic, each with a period of \( 2\pi \) radians, which means their graphs repeat every \( 2\pi \) radians. The sine function is odd, meaning it has rotational symmetry about the origin, satisfying the identity \( \sin(-\theta) = -\sin(\theta) \). This property results in a graph that is symmetrical with respect to the origin. Conversely, the cosine function is even, displaying mirror symmetry about the y-axis, as illustrated by the identity \( \cos(-\theta) = \cos(\theta) \). This symmetry results in a cosine graph that is identical on either side of the y-axis.
The tangent function, with a period of \( \pi \) radians, is also odd like the sine function. Its graph shows rotational symmetry about the origin, adhering to the identity \( \tan(-\theta) = -\tan(\theta) \). However, unlike sine and cosine, the tangent function exhibits vertical asymptotes at odd multiples of \( \frac{\pi}{2} \), where the function approaches infinity. These symmetry properties and periodic behaviors are fundamental in understanding the trigonometric functions and their applications in various mathematical and real-world contexts.
How do you teach this topic? Do you have any tips or suggestions for other teachers? It is always useful to receive feedback and helps make these free resources even more useful for Maths teachers anywhere in the world. Click here to enter your comments.