Topics: Starter | Algebra | Functions | Puzzles

• Liam Kelsall, Holden Lane High
•  
• Can we see some answers please? Our best answer is ☆☆☆☆ .
• K Hudson, Drigg
•  
• Elegant starter with lots of fun ideas to explore. The kids soon arrived at ☆☆☆☆ and went from there!
• Akbar Ali, Whitefield Community School
•  
• Both ☆☆☆☆ and ☆☆☆☆ give ☆ ☆ . This is because ☆ ☆ + ☆ ☆ + ☆ ☆ + ☆ ☆ = ☆ ☆ and ☆ ☆ + ☆ ☆ + ☆ ☆ + ☆ ☆ = ☆ ☆ I believe these are the only two solutions due to the symmetry in the answers.
• Chelsea Maher, Harrytown High School Stockport
•  
• without being told the single digit integer ruler, Chelsea Maher came up with
  
  ☆ ☆
  ☆ ☆ . ☆
  
  Well done
• Mr Wilson, 2MW
•  
• Our Year ☆ class, (all of whom are brainboxes obviously!) have come up with ☆☆☆☆ , ☆☆☆☆ , ☆ ☆ ☆ ☆ , ☆☆☆☆ and ☆ ☆ ☆ ☆
• J Nicholl, Kellett School Hong Kong
•  
• A pupil in my class saw the pattern that the apple digits must add up to ☆ .
• Miss Hickman, Wildern
•  
• My year nines found that the combination ☆☆☆☆ was a solution not already listed
• Mrs Simpson and 4M4, Torry Academy, Aberdeen
•  
• David in ☆ M ☆ found this solution: ☆☆☆☆
• Yeah!, Catherine Potter
•  
• It was really fun! All my family and I did it.
• Mr Baker, Somerset, UK
•  
• My year ☆ class loved this starter
  Soon found that if apples were lettered
  a b
  c d
  then ☆ a+b+c= ☆ and b+c+ ☆ d= ☆
  Brilliant one thanks :).
• Lee, Adelaide
•  
• ☆ , ☆ , ☆ , ☆ and the mirror also work.
• Mr Peter Okurut, Benjamin Britten High School, Lowestoft
•  
• Using algebra,
  ☆ ☆
  ☆ ☆ .
• Mr Page & 7J1, John Ferneley College
•  
• ☆ J ☆ found out using a,b,c,d to stand for the apples that:-
  a + b + c + d = ☆
  a = d (Archie Herrick & Josh Leach).
• Mrs Gocht, Year 3
•  
• Also ☆☆☆☆ works, my class loved it and I have year ☆ , but it did take them awhile! bless there little hearts!
• Alistair Carratt, Sanderson High School
•  
• I work in an ASN school. My S ☆ B class tried this puzzle and one pupil found ☆☆☆☆ and another found ☆☆☆☆ . This led us to find a pattern - all numbers must add up to ☆ and the grid must have symmetry across the diagonals. We found ☆ ☆ solutions.
• Mrs Ward, Cleeve School
•  
• We found out that ☆ ☆
  ☆ ☆ is a possible of ☆ ☆ .
• 6T, Widford Lodge
•  
• We found ☆☆☆☆ and ☆ ☆ ☆ ☆ too. It was good fun!
• Mrodwyer, Churchdown School Glos Year 8
•  
• Year ☆ pupils did this ☆ minute starter and arrived at all the previous answers already posted. very sharp class. and would like to add ☆ ☆ ☆ ☆ .
• Mrs. W And 1C1, Torry Academy, Scotland
•  
• We found, ☆☆☆☆ by Donnelly and ☆☆☆☆ by Natasha then noticed they added to ☆ . Great!
• Year 6M, Garswood Primary
•  
• We tried this in staff meeting verses our year sixes. Year Six got the answer quicker than some of our teachers!
• Mr J Saye, 8
•  
• Castle Rock High School Coalville
  My Set ☆ Year ☆ class had ☆ minutes to complete this task, one girl came up with another way to get ☆ ☆ in the lemon:
  ☆☆☆☆
  Well done to RMc!!!
  Mr Saye.
• Gr 12 Advanced Functions, Port Hope, Ontario, Canada
•  
• Lemon Number ☆ ☆
  ☆ ☆
  ☆ ☆
  also works.
• Roy Froud, Bournemouth
•  
• I would like to ask students if they could solve the problem sent home from school to my ☆ -year-old great nephew:
  a b
  c d
  
  In the grid shown, replace letters a, b, c, d, by ☆ different numbers from ☆ to ☆ so that adding the two rows and two columns together sums to ☆ ☆ ☆ .
  Example:
  ☆ ☆
  ☆ ☆
  
  ☆ ☆ + ☆ ☆ + ☆ ☆ + ☆ ☆ = ☆ ☆ ☆
  See if you can find the ☆ ☆ other combinations that work
  In all the above, b and c are interchangeable, leading to another ☆ ☆ solutions, although, as has been pointed out to me by my nephew, this amounts to transposing rows and columns.
  For fun, prove that d = a + ☆ and that a + b + c + d = ☆ ☆
  Roy Froud
  Voluntary Maths Tutor.
• Lars, Norway
•  
• Why does the answers not show any solutions where an apple has the number ☆ ?
  I used this way to solve the problem:
  The apples are labeled:
  a b
  c d
  the oranges are
  e = ☆ ☆ *a + ☆ *b
  f = ☆ ☆ *c + ☆ *d
  g = ☆ ☆ *a + ☆ *c
  h = ☆ ☆ *b + ☆ *d
  the lemon is then the toal sum:
  lemon = e + f + g + h
  = ☆ ☆ *a + ☆ ☆ *(b + c) + ☆ *d (after doing some algebra)
  Then it's easy to see alle the solutions which adds up to ☆ ☆ . you can start with saying that we put a= ☆ , this means that to get ☆ * ☆ ☆ , we must have that b+c= ☆ . In this case, the lemon will always be ☆ ☆ , and we must put d= ☆ . This means that every combination of:
  a, d = ☆ and b + c = ☆
  will give ☆ ☆ . There are ☆ different combinations of this.
  Next solution can be found by setting a= ☆ . we then need to add tens up to ☆ ☆ , which can only be done if b+c = ☆ . If so, d must be equal to ☆ . This gives:
  a, d = ☆ and b + c = ☆
  Every combination of this gives a lemon with ☆ ☆ , there are ☆ different combinations
  In fact, we must always have that a = d. There rest of the solutions are:
  a, d = ☆ and b + c = ☆ . This solution has ☆ different combinations
  a, d = ☆ and b + c = ☆ . This solution has ☆ different combinations
  a, d = ☆ and b + c = ☆ . This solution has only one combinations.
  In totale, there are therefore ☆ ☆ different ways to get the lemon number ☆ ☆ .
• Ella, St.James CEC Primary
•  
• Me and Mr.Wealend all came up with ☆☆☆☆ but Mr.Wealend said we had to get ☆ ☆
  and the apples can't add up to ☆ . We came up with ☆ ☆ ☆ ☆ and ☆ ☆ ☆ ☆ but they add up to ☆ .We sill can't get it.I found out that the apples had to go together to a two digit number which comes up in the orange then add together to get the number in the lemon.
• Sarah, St James Ptimary
•  
• ☆ ☆
  ☆ ☆
  Is a answer but we are trying to do it without it adding up to ☆ . It is a very hard problem but we like to do challenging problems.
• Ben L, St James' Primary
•  
• I found that the numbers in the apples have to add up to ☆ and if you can find one that does not add up to ☆ .........your very clever.
• Sarah And Molly, Mr Waelend's Maths Group
•  
• It doesn't work unless the number add up to ☆ . We tried putting ☆ in all the boxes, and then added one to it until it made ☆ ☆ . The one that we got up to in the end was ☆ ☆ Our headteacher set us a challenge of finding ☆ ☆
  ☆ ☆ .
  without the numbers in the apples adding up to ☆ .We think it is impossible. We will keep trying though.
• Ben, St James
•  
• I found the ☆ ☆ ☆ ☆ solution quite quickly and then found others like ☆☆☆☆ and ☆ ☆ ☆ ☆ however we rialised that all these combination's digits add up to ☆ so to try and discover if you can do it where the digits don't add up to ☆ we worked out what happened when we changed each number:
  top left: +/- ☆ ☆ top right:+/- ☆ ☆
  bottom left:+/- ☆ ☆ bottom right:+/- ☆
  Once I had found this information I tried to make ☆ ☆ by multiplying these different numbers by digits that when added don't make ☆ I couldn't find any without going in to decimals (which the thing dosn't allow).
• Alex+Conall, Mr Waelends Maths Group
•  
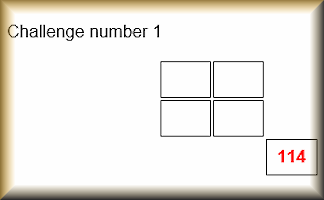
• We started off by doing ☆ ☆ ☆ ☆ then we started trying to do nunbers that didn't equal ☆ and worked out that you needed to make numbers that made the numbers in the oranges equal ☆ ☆ .
• Mr W, St James Ce School
•  
• We found the answer ☆ ☆ ☆ ☆ very quickly and felt extremely pleased with ourselves, so we set ourselves the challenge of trying to find other solutions. We found a number of them and discovered that the total on the apples always seemed to come to ☆ .
  We set out to try and find a solution that didn't add up to ☆ . In order to do this, we tried to find out the effect of each apple on the number on the lemon.
  In doing this, we soon discovered that the apple in the top left hand corner increased the lemon by ☆ ☆ when it was increased by one. We could see that this was because it was used as the tens digit twice in making the lemon number. The bottom right hand corner apple was only used as the units digit twice so added ☆ to the lemon when the number in it was increased by ☆ . The remining two apples were used once as a ten and once as a unit, so they added ☆ ☆ to the lemon when they were increased by ☆ .
  Suddenly the problem becomes much simpler. How can we make ☆ ☆ by adding ☆ ☆ s, ☆ ☆ s and ☆ s?
  We felt a little frustrated that we couldn't use numbers larger than ☆ or decimals. If we could then we could simply put ☆ ☆ in the bottom right hand corner. However, even ☆ + ☆ = ☆ !
  We were split in our minds about whether there was a solution or not.
  I tried by starting with no apples in the TL. To reach ☆ ☆ I could share ☆ apples between the BL and TR apples and leave ☆ in the BR apple. But this would add up to ☆ !
  If I only spread ☆ apples between the two corners it would leave me ☆ ☆ to make up with the BR corner. I can't make ☆ ☆ as I need an even number. Every number in the BR corner adds ☆ to the lemon.
  So I need to spread ☆ apples between the TR and BL corners, leaving ☆ ☆ to be added. I can only do this by adding ☆ ☆ to the BR corner. Too many.
  In carrying on in this way, we could find no combination of numbers that didn't add to ☆ !
• Rachel, St James' CEC Primary School
•  
• We found ☆☆☆☆ and ☆ ☆ ☆ ☆ like everyone else but, as we thought it was a bit too easy, we decided to find one that didn't add up to ☆ so here is what we found:
  I worked out that every time you change the top left apple by ☆ (up or down) the lemon increases or decreases by ☆ ☆ . When you increase or decrease the bottom right apple by ☆ (up or down) the lemon increases or decreases by ☆ . Then the top right apple, if changed by one (up or down) causes the lemon to decrease or increase by ☆ ☆ . Ditto for the bottom left apple.
  I am pretty sure it is impossible unless you can use decimal points and double digit numbers.
• Matthew, 3D, Craigslea State School
•  
• I found five possibilities;
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆ .
• Tc, Thailand
•  
• I labelled a b c d
  and then found that ☆ ☆ = ☆ ( ☆ ☆ a + b) + ☆ ☆ (b + c)
  so ☆ ☆ = ☆ ☆ + ☆ ☆
  or ☆ ☆ = ☆ ☆ + ☆ ☆
  or ☆ ☆ = ☆ ☆ + ☆ ☆
  see table below.. I got ☆ ☆ combinations for a b c d
  a d ☆ ☆ a + b b c b + c
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆
  ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆ ☆ .
• 5HC, The Beacon
•  
• This was fun!
  Manhar started us off by figuring that one particular digit works in all four slots.
  We ended up working out that there were ☆ ☆ ways of doing it (and ☆ ☆ if you allowed negative single-digit integers!). In between, Nayan spotted that the digits added up to ☆ , which really helped us, as did the realisation that the top right and bottom left apples always had the same sum.
• Roy Froud, Bournemouth
•  
• To all who have answered my original question, it is implied by the separate letters a b c d that they are all different integers. so there are only the ☆ ☆ or so answers I listed. Not sure about ☆ as an integer. Not in my book but someone will argue!
• Maths Is Fun Set, United Kingdom
•  
• We are a year ☆ maths group. We did pretty well. We enjoyed it. Our teacher helped us a little bit. This makes our brains work.

How did you use this starter? Can you suggest how teachers could present or develop this resource? Do you have any comments? It is always useful to receive feedback and helps make this free resource even more useful for Maths teachers anywhere in the world.
Click here to enter your comments.

Previous Day | This starter is for 12 January | Next Day