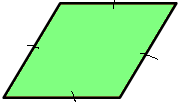
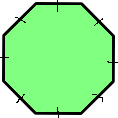
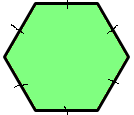
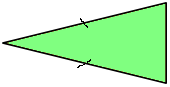
Show how many lines of symmetry each polygon has by dragging to the plaques beneath each shape.
|
|
|
|
|
|
|
|
|
|
|
|
Two
None
Two
Four
One
Eight
Six
None
One
One
Five
None
Printable Version Polygon People Polygon Properties Polybragging
|
|
||
Transum.orgThis web site contains over a thousand free mathematical activities for teachers and pupils. Click here to go to the main page which links to all of the resources available. Please contact me if you have any suggestions or questions.
|
More Activities: |
|
|
Mathematicians are not the people who find Maths easy; they are the people who enjoy how mystifying, puzzling and hard it is. Are you a mathematician? Comment recorded on the 1 February 'Starter of the Day' page by Terry Shaw, Beaulieu Convent School: "Really good site. Lots of good ideas for starters. Use it most of the time in KS3." Comment recorded on the 14 September 'Starter of the Day' page by Trish Bailey, Kingstone School: "This is a great memory aid which could be used for formulae or key facts etc - in any subject area. The PICTURE is such an aid to remembering where each number or group of numbers is - my pupils love it! |
Each month a newsletter is published containing details of the new additions to the Transum website and a new puzzle of the month. The newsletter is then duplicated as a podcast which is available on the major delivery networks. You can listen to the podcast while you are commuting, exercising or relaxing. Transum breaking news is available on Twitter @Transum and if that's not enough there is also a Transum Facebook page. |
|
Featured ActivityNumber Crunch Saga A lively numeracy game requiring you to align three numbers to create the given target sum or product. There are five levels to this online game and a virtual Transum Trophy available for each level. |
Numeracy"Numeracy is a proficiency which is developed mainly in Mathematics but also in other subjects. It is more than an ability to do basic arithmetic. It involves developing confidence and competence with numbers and measures. It requires understanding of the number system, a repertoire of mathematical techniques, and an inclination and ability to solve quantitative or spatial problems in a range of contexts. Numeracy also demands understanding of the ways in which data are gathered by counting and measuring, and presented in graphs, diagrams, charts and tables." Secondary National Strategy, Mathematics at key stage 3 |
|
Go MathsLearning and understanding Mathematics, at every level, requires learner engagement. Mathematics is not a spectator sport. Sometimes traditional teaching fails to actively involve students. One way to address the problem is through the use of interactive activities and this web site provides many of those. The Go Maths main page links to more activities designed for students in upper Secondary/High school. | ||
Teachers | ||
|
If you found this activity useful don't forget to record it in your scheme of work or learning management system. The short URL, ready to be copied and pasted, is as follows: |
Alternatively, if you use Google Classroom, all you have to do is click on the green icon below in order to add this activity to one of your classes. |
It may be worth remembering that if Transum.org should go offline for whatever reason, there are mirror site at Transum.info that contains most of the resources that are available here on Transum.org. When planning to use technology in your lesson always have a plan B! |
|
Do you have any comments? It is always useful to receive feedback and helps make this free resource even more useful for those learning Mathematics anywhere in the world. Click here to enter your comments. |
||
Transum,
Wednesday, September 3, 2014
"Knowing the names of the polygons is only the start. Each shape has its own properties which define it. There are families of shapes and some shapes can be identified with more than one name. The next step is to show your knowledge of Polygon Properties with our interactive matching activity."
Luke Farrand, Rosmini College
Friday, October 19, 2018
"It has recently come to my knowledge when attempting one of your activities under Topic - Geometry - Activities - Polygons - Rotational Symmetry there is an error in this task.
If I am not mistaken all shapes have a rotational symmetry of at least one, however in this task it shows shapes with a rotational symmetry of one are considered none.
This little mistake may be enough to make someone fail their exam or fall one excellent credit short of endorsement in this wrong teaching. Therefore I recommend you change it before an unfortunate student suffers that fate."
Transum,
Friday, October 19, 2018
"Dear Luke,
Thank you so much for taking the time to make a comment about rotational symmetry.
Firstly I must say that sometimes in Mathematics everyone does not agree on certain definitions. For many years the UK defined a billion as 1012 while the US defined it as 109. Some people believe that zero is a member of the set of natural numbers while others do not. I’m afraid rotational symmetry may also attract mixed opinions.
According to all of the online references and textbooks I have seen there is no such thing as rotational symmetry of order one. As Wikipedia states: "Note that '1-fold' symmetry is no symmetry (all objects look alike after a rotation of 360°)".
If you have a reference that disagrees with this way of thinking please let me know.
I really would appreciate hearing any further comments you may have about the activities on the Transum website.
Best wishes "
Tony, VAS
Wednesday, September 1, 2021
"Dear Transum and Luke,
I agree with luke's statement here. According to BBC Bitesize, it is clearly stated that the minimum number of rotational symmetry is 1.
I would love to hear a statement from all of you!
[Transum: Thanks very much Tony for the link showing that respected websites disagree with the definition. I have now taken time to do some more research and I think the balance of opinion agrees with you so. Having said that I don't think the word 'none' on the draggable card is wrong. Perhaps 'zero' would be wrong but I think 'none' is technically correct isn't it?]"
Paul Hall, Private Tutor
Wednesday, December 28, 2022
"Dear Sir/Madam,
Many thanks for your website I teach online, and I don't know what I would do without Transum.
My comment concerns Rotational Symmetry, of order 1, as I see it.
A rectangle has RS of order 2. It looks the same two ways around. One of these two ways includes the unrotated shape.
A scalene triangle has RS of order 1. It looks the same one way around. This is the unrotated shape.
To say that it has RS of order 0 is to say that we ignore the original orientation, which we do not do in the case of the rectangle, otherwise the rectangle has RS of order 1.
This makes a special case of the shape, and of the number 1.
In one case, 1 = 1, and in the other case, 1 = 0.
This is, of course, nonsense.
I look forward to your reply.
I understand your comments about zero being a natural number, or not, and the definition of a billion. However, I have never seen exam questions which would catch people out if they made the wrong choice.
These are matters of choice you can decide that 0 is a natural number, or not, as you wish. You can decide on the definition of a billion, as you wish, though I deprecate words like zillions, and wish that people would powers of 10.
Respectfully yours: P C Hall."
Transum,
Thursday, December 29, 2022
"Dear Paul, thank you so much for your comments. I agree that we should not say that a scalene triangle has rotational symmetry of order zero or one. I believe the best statement is that it has no rotational symmetry. It’s a special case just like the word group. You could have a group of ten people you could have a group of three prople but you would not describe one person as a group!"