Number Sequences 1

What is the 7th:
a) Odd number; 13
b) Square number; 49
c) Prime number. 17

Factors

Find all the factors of:

28

1, 2, 4, 7, 14, 28.

Multiples

Subtract the 4th from the 7th multiples of:

7

21

Polygons

What are the names of regular polygons with:
a) four sides;
b) five sides;
c) six sides.

Square, Pentagon and Hexagon (all regular)

Rounding (3sf)

Round to three significant figures:
a) 74.58; 74.6
b) 267312; 267000
c) 13; 13.0
d) 0.001395; 0.00140

Area of a Triangle

Find the area of a triangle that has a base of 7cm and a height of 11cm.

38.5cm²

Area of a Trapezium

Find the area of a trapezium that has a base of 12cm, a height of 8cm and a top (parallel to base) of 4cm. 64cm²

Fractions (Adding)

Evaluate:

\( \frac{1}{2} + \frac{4}{6}\) \(= 1\frac{1}{6}\)

Fractions (Multiplying)

Evaluate:

\( \frac{2}{4} × \frac{6}{7}\) \(= \frac{3}{7}\)

Fractions (Dividing)

Evaluate:

\( \frac{3}{5} ÷ \frac{7}{6}\) \(= \frac{18}{35}\)

Circle (Vocabulary)

Name the red part.

Venn Diagrams

Describe the red region.

Shape Formulas

What is the formula?

Formulas (Advanced)

What is it?

Fraction to Percentage

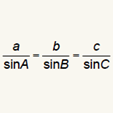
Convert this fraction to a percentage to 3 significant figures.

\( \frac{4}{6}\) \(= 66.7\)%

Circle Area

Find the area of a circle that has a radius of 3cm. Give your answer to three significant figures.

28.3cm²

Circle Circumference

Find the circumference of a circle that has a radius of 11cm. Give your answer to three significant figures.

69.1cm

Decimals (Adding)

Calculate the value of:

8.8 + 6.8

= 15.6

Decimals (Subtracting)

Calculate the value of:

6.2 − 4.7

= 1.5

Decimals (Multiplying)

Calculate the value of:

2.6 × 2.6

= 6.76

Decimals (Dividing)

Calculate the value of:

100.8 ÷ 14

= 7.2

Indices (Simple)

What is the value of:

3³

= 27

Indices (Advanced)

What is the value of:

\(5^{-1}\)

\(= \frac{1}{5}\)

Basic Addition

Calculate the value of:

38 + 84

= 122

Basic Subtraction

Calculate the value of:

53 − 25

= 28

Basic Multiplication

Calculate the value of:

75 × 25

= 1875

Basic Division 2

Calculate the value of:

1155 ÷ 15

= 77

Percentage (Of)

Find the value of:

60% of 140

= 84

Standard Form 1

Find the value of:

3.03 × 10⁴

= 30300

Highest Common Factor

Find the highest common factor of thirty two and eight.

= 8

Times Tables (2-5)

Times Tables (2-12)

Times Tables (2)

Times Tables (3)

Times Tables (4)

Times Tables (5)

Times Tables (6)

Times Tables (7)

Times Tables (8)

Times Tables (9)

Times Tables (12)

Fractions (Equivalent)

Write this fraction in its simplest form:

\( \frac{12}{24}\) \(= \frac{1}{2}\)

Fractions (Mixed)

Evaluate:

\( 1\frac{4}{5} − \frac{6}{7}\) \(= \frac{33}{35}\)

Pythagoras

Find BC if AB = 5.8m and AC = 6.9m. 9.01m

Trigonometry (Angle)

Find angle BCA if AC = 5.3m and BC = 6.9m. 39.8^o

Trigonometry (Side)

Find AB if angle ABC = 56^o and BC = 3.7m. 2.07m

Roman Numerals (1-12)

Give your answer in Roman numerals.

²

Roman Numerals (60-100)

Give your answer in Roman numerals.

²

Roman Numerals (Large)

Give your answer in Roman numerals.

²

Fraction to Decimal

Convert this fraction to a decimal.

\( \frac{1}{5}\) \(= 0.2\)

Decimal to Fraction

Convert this decimal to a fraction.

\(0.29\) = \( \frac{29}{100}\)

Percentage (Increase)

Increase £20 by 35%

£27

Lowest Common Multiple

What is the lowest common multiple of twelve and twenty one.

= 84

Sequence (Arithmetic)

3,12,21,30,39...

Find the:
a) next term; 48
b) n^th term; 9n - 6
c) term number 33; 291

Sequence (Geometric)

4,12,36,108,324...

Find the:
a) next term; 972
b) n^th term; 4 × 3^n-1
c) term number 8; 8748

Interest (Simple)

If £160 is invested for 4 years with a simple interest rate of 5%, find the amount of interest earned. £32.00

Interest (Compound)

If £100 is invested with an interest rate of 6% compounded annually, find the value of the investment after 7 years. £150.36

Currency Exchange

If £1 is worth $1.31, convert:

a) £100 to dollars; $131.00

b) $220 to pounds; £167.94

Coordinates (Midpoint)

What are the coordinates of the midpoint of the line joining:

\((-2,3) \text{ and } (8,13)\)

(3,8)

Gradient

What is the gradient of the line joining:

\((-4,7) \text{ and } (0,13)\)

\(\frac{3}{2}\)

Coordinates (Square)

Here are the coordinates of 3 vertices of a square, what are the coordinates of the 4^th?

\((1,4),(6,10),(-5,9)\)

(0,15)

Negative Numbers

a) 8 − 19 = -11
b) 8 × (-12) = -96
c) (12−20)(5−11) = 48
d) 96 ÷ (-12) = -8
e) (-12)² = 144

Substitution

If p = 6, q = 25 and
r = -11 evaluate:

a) 2q − p = 44
b) pq + r = 139
c) p² − 5q - r = -78

Equations (Type 1)

Solve:

\(2x = 4\)

\(x = 2\)

Equations (Type 2)

Solve:

\(5x +8= 53\)

\(x = 9\)

Equations (Type 3)

Solve:

\(10x +6= 5x + 31\)

\(x = 5\)

Equations (Type 4)

Solve:

\(3(5x +5)+12= 102\)

\(x = 5\)

Equations (Type 5)

Solve:

\(6(4x + 3)= 4(5x + 2)\)

\(x = -2.5\)

Equations (Simultaneous 1)

Solve:

\(3x-5y = -4\)
\(2x-5y = -6\)

\(x = 2, y = 2\)

Equations (Simultaneous 2)

Solve:

\(2x-2y = -4\)
\(7x+8y = 76\)

\(x = 4, y = 6\)

Equations (Simultaneous 3)

Solve:

\(2x+7y = -48\)
\(5x-3y = 3\)

\(x = -3, y = -6\)

Sets (Union)

Find the union of:

{2,4,6,8,10} and
{1,3,6,10,15}

{1,2,3,4,6,8,10,15}

Sets (Intersection)

Find the intersection of:

{6,7,8,9,10} and
{3,4,5,6,7,8}

{6,7,8}

Bearings

A plane flies from point A to point B on a bearing of 242^o. What bearing would it return on from B to A? 062^o

Probability

A number is picked at random from the set

{2,3,5,7,11,13}

what is the probability it is even? \(\frac16\)

BIDMAS

Evaluate:

6² − 8 × 7 + 7

-13

Simplify

Simplify the following by collecting like terms:

\(5a−5−4a−5\)

\(a-10\)

Ratio

Divide 132 in the ratio

9:2

108 and 24

Graph Linear (1)

Draw a rough sketch of the graph of:

\(y=2x-2\)

Gradient 2
y intercept -2

Prime Factors

Express the following number as the product of prime numbers:

30

2 x 3 x 5

Percentage (Reverse)

In a sale an item costs £48 after a 20% reduction. What was the original price?

£60

Averages

Find the mean, mode, median and range of the following:

2,4,6,8,10

Mean = 6, no mode,
median = 6 and range = 8

Time (Analogue)

What time is this?

Time (Digital)

Sketch a clock face:

Decimals (Recurring)

Write the following recurring decimal as a fraction in its lowest terms.

0.868686... \(\frac{86}{99}\)

Percentage (Decrease)

Decrease £180 by 25%

£135

Brackets (Linear)

Expand:

\(7(3x-3)\)

\(21x-21\)

Brackets (Quadratic)

Expand:

\((3x+3)(2x-2)\)

\(6x^2-6\)

Factorise (Linear)

Factorise:

\(8x-6\)

\(2(4x-3)\)

Factorise (Quadratic 1)

Factorise:

\(x^2-2x-3\)

\((x+1)(x-3)\)

Factorise (Quadratic 2)

Factorise:

\(5x^2+7x-6\)

\((x+2)(5x-3)\)

Circle Theorems

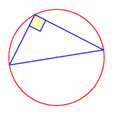
Which theorem?

Standard Form 2

Find the value of:

3.95 × 10^-2

= 0.0395

Standard Form 3

Write in standard form:

254000

= 2.54 × 10⁵

Standard Form 4

Write in standard form:

0.0518

= 5.18 × 10^-2

Sequence (Quadratic)

Find the n^th term:

\(12, 18, 26, 36, 48, \)

\(n^2+3n+8\)

Standard Form 5

Multiply 6 × 10²
by 7 × 10³ and give the answer in standard form.

= 4.2 × 10⁶

Equations (Quadratic 1)

Solve:

\(x^2+x-6= 0\)

\(x = 2\) and \(-3\)

Equations (Quadratic 2)

Solve this equation giving the solutions to 3 significant figures:

\(3x^2+x-5 = 0\)

\(x = 1.14\) and \(-1.47\)

Polygon Angles

What is the size of each exterior angle of a regular decagon?

36°

Change The Subject

Make \(d\) the subject of the formula
$$e=\frac{2d-1}{3}$$

$$d=\frac{3e+1}{2}$$

Basic Division 1

Calculate the value of:

2728 ÷ 4

= 682

Number Sequences 2

What is the 10th:
a) Cube number; 1000
b) Triangular number; 55
c) Fibonacci number. 55

Square Numbers

What is the square root of

16

4

Prime Numbers

What are the three largest prime numbers less than
31

29, 23, 19

Last Lesson

Write down something you learnt in the previous mathematics lesson.

Last Week

Write down something you learnt in one of the mathematics lessons last week.

Angles

Calculate \(x\).

Decimals (Ordering)

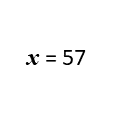
Write down these numbers: 0.8, 0.88, 8.08, 8.88, 8, 8.8, 0.08, in ascending order.
0.08, 0.8, 0.88, 8, 8.08, 8.8, 8.88,

Lengths (Ordering)

Write down these lengths: 18mm, 1.8m, 1.08m, 17cm, 107cm, 1.7cm, in order.
1.7cm, 18mm, 17cm, 107cm, 1.08m, 1.8m,

Capacities (Ordering)

Write down these capacities: 200ml, 18cl, 173ml, 21cl, 17cl, 18ml, in order.
18ml, 17cl, 173ml, 18cl, 200ml, 21cl,

Angles with Parallels 1

e = 69

Angles with Parallels 2

c = 78

Rounding (1sf)

Round to one significant figure:
a) 15.41; 20
b) 476203; 500000
c) 82; 80
d) 0.00502; 0.005

Estimation

Round each value to one significant figure to make an estimate:$$61 + 2.7 \times 93$$\(60 + 3 \times 90 = 330\)

Pie Charts (1)

A pie chart shows the colours of 18 books. What sector angle represents the
16 red books?
320°

Graph Linear (2)

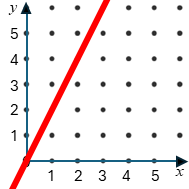
What is the equation?

Pie Charts (2)

A pie chart shows the colours of 9 hats. How many green hats are represented
by a sector
angle of 80°?
2

Surds (1)

Simplify:
$$\sqrt{20}$$
\(2\sqrt{5}\)

Surds (2)

Simplify:
$$\dfrac{3}{4\sqrt{2}}$$\(\frac{3\sqrt{2}}{8}\)

Surds (3)

Simplify:
$$\dfrac{5}{3 - \sqrt{2}}$$\(\frac{15 + 5\sqrt{2}}{7}\)

Speed, Distance, Time

What distance is covered by a jogger running at 20 km/h for 6 hours?
👟120 km

Factorising by Grouping

Factorise:

\(5ab - 10a - 2b + 4\)

\((5a-2)(b-2)\)

Algebraic Fractions

Simplify:

\(\dfrac{2}{x+1} - \dfrac{1}{x-1}\)

\(\dfrac{x-3}{x^{2}-1}\)

Equations with Fractions

Solve:

\(\dfrac{7}{x+2}-\dfrac{x+1}{2x}=1\)

\(x=2 \text{ or}\ x=\frac{1}{3}\)