|
|
CoordinatesA self marking exercise on identifying and naming coordinates (Four quadrants). |
Each square on the grid represents one unit. Type your answers without spaces but remembering the brackets and the comma like this (3,4)
The black lines are the axes and they cross at the origin.
|
What are the coordinates of the purple point? |
|
|
What are the coordinates of the lime point? |
|
|
What are the coordinates of the brown point? |
|
|
What are the coordinates of the yellow point? |
|
|
What are the coordinates of the pink point? |
|
|
What are the coordinates of the maroon point? |
|
|
What are the coordinates of the orange point? |
|
|
What are the coordinates of the red point? |
|
|
What are the coordinates of the blue point? |
|
|
What are the coordinates of the green point? |
|
InstructionsTry your best to answer the questions above. Type your answers into the boxes provided leaving no spaces. As you work through the exercise regularly click the "check" button. If you have any wrong answers, do your best to do corrections but if there is anything you don't understand, please ask your teacher for help. When you have got all of the questions correct you may want to print out this page and paste it into your exercise book. If you keep your work in an ePortfolio you could take a screen shot of your answers and paste that into your Maths file. |
||
|
|
||
|
More Activities: |
|
|
Mathematicians are not the people who find Maths easy; they are the people who enjoy how mystifying, puzzling and hard it is. Are you a mathematician? Comment recorded on the 1 February 'Starter of the Day' page by Terry Shaw, Beaulieu Convent School: "Really good site. Lots of good ideas for starters. Use it most of the time in KS3." Comment recorded on the 2 April 'Starter of the Day' page by Mrs Wilshaw, Dunsten Collage,Essex: "This website was brilliant. My class and I really enjoy doing the activites." |
Whose Idea Was This?Did you enjoy doing this 'Coordinates' activity? Are you curious about who originally came up with this idea in Maths? Discover more about one of the mathematicians who is associated with this concept. 
|
|
Featured ActivityNewsletter The Transum Newsletter for December 2024 has just been published. Click on the image above to read about the latest developments on this site and try to solve the puzzle of the month. You can read the newsletter online or listen to it by downloading the podcast. |
AnswersThere are answers to this exercise but they are available in this space to teachers, tutors and parents who have logged in to their Transum subscription on this computer. A Transum subscription unlocks the answers to the online exercises, quizzes and puzzles. It also provides the teacher with access to quality external links on each of the Transum Topic pages and the facility to add to the collection themselves. Subscribers can manage class lists, lesson plans and assessment data in the Class Admin application and have access to reports of the Transum Trophies earned by class members. If you would like to enjoy ad-free access to the thousands of Transum resources, receive our monthly newsletter, unlock the printable worksheets and see our Maths Lesson Finishers then sign up for a subscription now: Subscribe |
|
Go MathsLearning and understanding Mathematics, at every level, requires learner engagement. Mathematics is not a spectator sport. Sometimes traditional teaching fails to actively involve students. One way to address the problem is through the use of interactive activities and this web site provides many of those. The Go Maths page is an alphabetical list of free activities designed for students in Secondary/High school. Maths MapAre you looking for something specific? An exercise to supplement the topic you are studying at school at the moment perhaps. Navigate using our Maths Map to find exercises, puzzles and Maths lesson starters grouped by topic. | ||
Teachers | ||
|
If you found this activity useful don't forget to record it in your scheme of work or learning management system. The short URL, ready to be copied and pasted, is as follows: |
Alternatively, if you use Google Classroom, all you have to do is click on the green icon below in order to add this activity to one of your classes. |
It may be worth remembering that if Transum.org should go offline for whatever reason, there is a mirror site at Transum.info that contains most of the resources that are available here on Transum.org. When planning to use technology in your lesson always have a plan B! |
|
Do you have any comments? It is always useful to receive feedback and helps make this free resource even more useful for those learning Mathematics anywhere in the world. Click here to enter your comments. |
||
Don't wait until you have finished the exercise before you click on the 'Check' button. Click it often as you work through the questions to see if you are answering them correctly. You can double-click the 'Check' button to make it float at the bottom of your screen.
Answers to this exercise are available lower down this page when you are logged in to your Transum account. If you don’t yet have a Transum subscription one can be very quickly set up if you are a teacher, tutor or parent.
Close
Max, Australia
Tuesday, August 8, 2017
"I would just like to point out that in your section called "First Quadrant", which you advertised that it had questions that consisted of questions from the first quadrant, had questions that involved coordinates that were placed on the axis, which are not technically in the first quadrant. The class got very annoyed and would appreciate you changing this. Thank you."
Transum,
Tuesday, August 8, 2017
"Thanks for your observation Max. If the first quadrant is the region where both the x and y coordinates are positive then any point on either of the axes should not be included as zero is not a positive number. You are absolutely right.
Having realised that I would like to suggest that the inclusion of points on the axes makes the exercise slightly more challenging and worthwhile. I hope you'll agree.
Now Levels 1 and 2 are purely first quadrant without zeros and Level 3 contains zeros and fractional coordinates.
Thanks again for your comments."
Transum,
Monday, December 10, 2018
"Some people have trouble remembering what the two numbers in a set of coordinates represent. They could be thought of as the route to the point from the origin. The first number is the x-coordinate and tells you how many units you go across to the right (negative numbers move you to the left). The second number tells you how many to move up (negative numbers move you down).
"
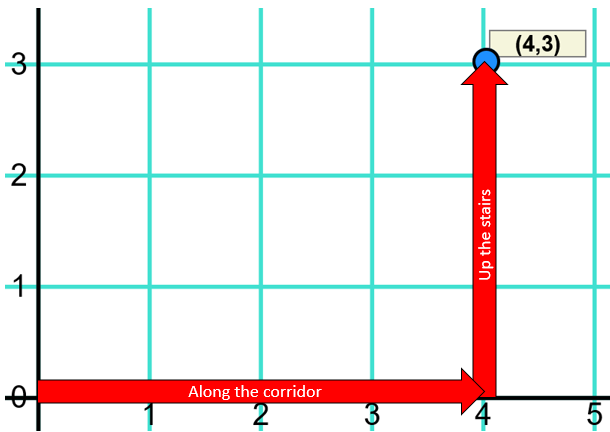The following saying helps you remember the order of the coordinates:
Along the corridor then up the stairs.
Greg, Wales
Monday, August 26, 2019
"Excellent resource, I use it all of the time! The only problem is that there is too much good stuff here!!"
AJ, UAE
Sunday, March 10, 2024
"Why do we need to write (5,6) instead of 56?
[Transum: Using this format helps distinguish between the two distinct values that define a point's location in space. If we were to write 56 instead of (5,6), it would not only contradict standard mathematical notation but could also lead to confusion, as 56 could be interpreted as a single number rather than a pair of coordinates.] "